- Tubelator AI
- >
- Videos
- >
- Education
- >
- Introduction to Trigonometry For Beginners: Understanding SOH CAH TOA and Right Triangle Trigonometry
Introduction to Trigonometry For Beginners: Understanding SOH CAH TOA and Right Triangle Trigonometry
Learn the basics of trigonometry for beginners, including understanding the SOH CAH TOA expression and right triangle trigonometry. Explore the concepts of opposite, adjacent, and hypotenuse sides, Pythagorean theorem, and the sine, cosine, and tangent functions. Start mastering trigonometry today!
Video Summary & Chapters
No chapters for this video generated yet.
Video Transcript
Have you ever heard of the expression SOH CAH TOA?
What do you think this expression means? In this lesson, we're going to focus on right triangle trigonometry.
Let's say if this is the angle theta.
Now there's three sides of this triangle that you need to be familiar with.
Opposite to theta, this is the opposite side, and next to the angle theta is the adjacent side.
and across the box or the right angle of the triangle which is the hypotenuse.
That's the longer side of the triangle. Now if you recall this is a b and c.
The Pythagorean theorem applies to right triangles. a squared plus b squared is
equal to c squared. We're not going to focus on that too much but just be
familiar with that equation.
Now let's talk about the six trig functions in terms of sine, cosine, tangent, opposite, adjacent, and hypotenuse.
Sine theta,
according to Sohcahtoa, S is for sine, O is for opposite, H is for hypotenuse.
Sine theta is equal to the opposite side
divided by the hypotenuse.
Cosine theta is
equal to the adjacent side
divided by the hypotenuse.
K is for cosine is adjacent over hypotenuse. And tangent theta, TOA, is equal to the opposite
side divided by the adjacent side. So that's the tangent ratio. It's opposite over adjacent.
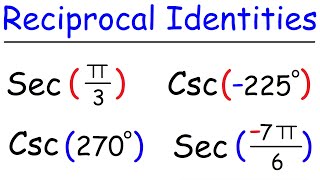
Now we know that cosecant is 1 over sine. So cosecant is basically hypotenuse divided
by the opposite side. You just need to flip this particular fraction. Secant is...
the reciprocal of cosine. So secant is going to be hypotenuse divided by the adjacent side.
Cotangent is the reciprocal of tangent. So if tangent is opposite of adjacent, cotangent
is adjacent divided by the opposite side. Now let's say if we're given a right triangle
and we have the value of two sides. Let's say this is 3 and this is 4 and here is the
angle theta. Find the missing side of this right triangle and then find the values of
all six trigonometric functions sine, cosine, tangent, secant, cosecant, cotangent. Now
to find the missing side we need to use the Pythagorean theorem. A squared plus B squared
is equal to C squared. So A is 3, B is 4. And we got to find the missing side C which
as I've honours three squared is nine four
squared is 16. 9 plus 16 is 25. And if you take the square root of both sides, you can
see that the hypotenuse is 5. Now it turns out that there are some special numbers. There's
the 3, 4, 5 right triangle, the 5, 12, 13 right triangle, the 8, 15, 17 right triangle,
and the 7, 24, 25 right triangle. And any whole number ratios or multiples of these numbers will
also work. For example, if we multiply this by 2, we'll get 6, 8, 10. That can also work. Or,
if you multiply it by 3, you get the 9, 12, 15 triangle. If you multiply this one by 2,
So you get the 10, 24, 26 triangle.
Those are also special triplets.
with any right triangle. Now some other numbers that are less common but you
might see are the 9 40 41 triangle and the 11 60 61. So if you see some of these
numbers you can find their missing side quickly if you know them. So now let's
finish this problem. So what is the value of sine theta? So according to Sohcah
Toah we know that sine theta is equal to the opposite side divided by the adjacent
side and the part so s o h opposite to theta is four and hypotenuse is five so therefore sine
theta is going to be four divided by five now cosine theta is equal to the adjacent side divided
by the hypotenuse. So we said 4 is the opposite side, 5 is the hypotenuse,
and 3 is the adjacent side. So in this case it's going to be 3 divided by 5.
So that's the value of cosine. Now let's find the value of tangent.
Tangent theta, according to Toa, is equal to the opposite side divided by the adjacent side.


 Install Tubelator On Chrome
Install Tubelator On Chrome